Nick Rowe's example from 'The sense in which the stock of money is "supply-determined"'

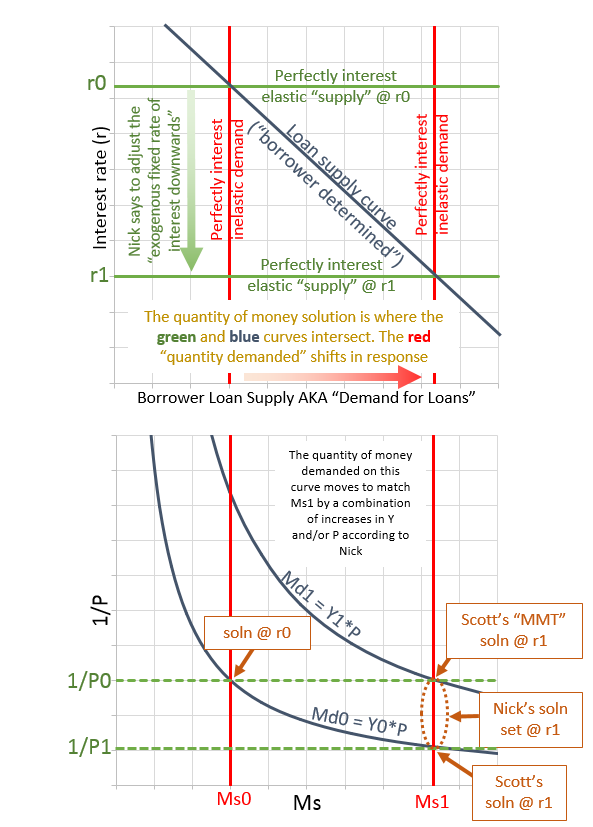
Figure 1: Diagram of Nick Rowe's example

In a post from Nick Rowe regarding the sense in which the stock of money is "supply-determined" he sketches out an example with the following definition for the money demand function:

Md=L(P,Y)=PY

He also mentions that:

By cutting the rate of interest, the central bank increases the quantity of loans from the central bank, which creates more money. Eventually P and/or Y will increase and the quantity of money demanded will increase in proportion to the quantity created.

Figure 1 is my attempt to sketch out what this example looks like. The items with "0" indicate the original values prior to the central bank's exogenous cut in interest rates from r0 to r1. Keep in mind that the central bank (CB) is the ONLY bank in this example: there are no commercial banks, and it only loans cash (so no deposits at all in this world) and it is compelled to lend to anyone because they are all good credit risks. I'll also assume that the bank is compelled to sell back to each individual borrower their debt on a dollar for dollar basis at the discretion of the borrower. Some of these assumptions may not be important for the logic of the post though!

Note that the blue downward sloping curve in the upper plot is the "supply curve for loans determined by borrowers" which Nick states is equivalent to "demand for loans by borrowers" in "normal language." He points this out in the comments in his previous post. Also note Md0 corresponds to Y0 and Md1 corresponds to Y1 (if P did not change from P0). However, it's possible also that Y does not change from Y0 and P instead changes from P0 to P1. In fact Nick implies that either or both P and Y could increase in this case which gives us the set of solutions for 1/P lying between the two blue money demand curves (Md0 and Md1) along the red supply curve on the right (Ms1).

Scott Sumner thinks that Nick must mean that where Md0 and Ms1 cross is the solution. He also claims that the MMT people would say that the solution is where Md1 and Ms1 cross. I think Nick is actually saying it can lie anywhere between those extremes.

Also note that in the upper plot the two horizontal green solid lines are the "perfectly-elastic supply functions" for money (each at a fixed interest rate, r0 and r1), and that the two vertical red lines in the upper chart are the two "perfectly interest inelastic" demand curves for  money. Assume the red vertical line on the left is Md0 and that it has already reached equilibrium to match the supply of money (Ms0 in the lower chart). After r is lowered from r0 to r1, then Ms0 goes to Ms1 in accordance with the borrower determined supply for loans curve in the upper plot. Then *eventually* the demand curve for money in the upper plot (still perfectly interest inelastic, and thus vertical) will move right to match the supply curve (Ms1) in the lower plot.

One more thought on the upper plot (with the CB "exogenously" determined interest rate on the y-axis). The solution where the downward sloping blue curve (borrower determined supply curve for loans) intersects the horizontal green "perfectly interest-elastic 'supply' curves for money." I've already noted how in "normal language" (according to Nick) the "supply curve for loans" is called "demand for loans." However, it's better described as a supply curve. But then we have two supply curves intersecting. But then it occurred me to make the following linguistic substitutions, and I think it all becomes a lot more clear:

Replace "loans" with "bonds."
Replace "borrowers" with "bond issuers."

Then the blue line's name makes more sense as a supply curve as we've noted. But also, the horizontal "supply for money" curves instead become "perfectly interest-elastic *DEMAND* curves for bonds at exogenously fixed rates of interest." So then we're back to quantity being determined in the usual way by a supply curve intersecting a demand curve. Hurray! I comment on that here on Nick's post.

The red perfectly interest-inelastic vertical demand for money curves in the upper plot do NOT determine the quantity of money, and instead follow the solution determined by the other two curves.

Note: Scott Sumner suggested in a comment that instead of 1/P on the y-axis in the lower plot, I should instead plot 1/NGDP = 1/(P*Y). If I did this, then there would only be a single downward sloping (rectangular hyperbola) blue Md curve and the solution set of Nick Rowe at Ms1 would collapse to a single point on that curve. I.e. Scott's solution and the "MMT" solution would be indistinguishable (which would probably not please Scott!).

Note that in the upper plot, the x-axis could be labeled "Quantity of loan-principal demanded/supplied (measured in dollars)."

With this later comment, Nick Rowe essentially establishes his solution set at r1 (in Figure 1's lower plot) to be the entire red ray labelled "Ms1"... it would be the small line segment if P and Y were precluded from decreasing.

Nick Rowe vs Scott Sumner: Different Answers to a Simple HPE Hypothetical

Figure 1: Nick Rowe's and Scott Sumner's solutions for P

This is a simple hypothetical that I feel get's to the core of concepts like the long term neutrality of money, the quantity theory of money (QTM), and the hot potato effect (HPE) which undergird much of Market Monetarist (MM) thought. If I'm not mistaken, Nick Rowe seems to think my hypothetical is a case where those concepts don't work, while Scott disagrees. Why do I care? Well if they don't work here, then what about our real situation does make them work? How can we be certain that they are indeed working, and if they are how do we know they are completely working?

Setup: This is basically the same as Scott Sumner's "cashless society" hypothetical (case 7) except with the added stipulation that there's just one commercial bank and that the reserve requirement is 0% (Scott didn't specify the number of commercial banks or the reserve requirement). In particular, we have:

1. Cashless society (no paper reserve notes and no coins).
2. A central bank (CB) which can buy and sell assets (open market operations (OMOs)).
3. A single commercial bank with a CB deposit (i.e. reserve deposit). This is the only existing CB deposit account.
4. The reserve requirement is 0%.
5. A non-bank private sector which holds deposits at the commercial bank.

Scott points out that in such a case base money consists only of bank reserves (as there's no cash) and thus reserves alone are the medium of account (MOA)*. Now for some important definitions:

P = average steady state price level in the private economy
Ms = reserve supply
Mdn = nominal reserve demand
Mdr = real reserve demand = Mdn/P

Scott Sumner and Mark A. Sadowski say that if Ms changes (e.g. through CB OMOs), then P will change in proportion. Nick Rowe says that in this case demand for reserves will change with Ms. Specifically he noted that if Ms goes to zero then demand for reserves will go to zero. If we take this to mean that demand is proportional to Ms, then P stays fixed regardless of the change in Ms.

The difference between Nick's and Scott's solution is illustrated in Figure 1 for two different values of Ms: we're assuming they start off the same at Ms = Ms0, but then the CB sells assets such that Ms = Ms1. The demand curve (Mdn) in Nick's case scales in proportion to Ms (this is accomplished by scaling Mdr). Nick Rowe says that my Figure 1 looks roughly right. Click here to see an animation.

My question for Nick is what is preventing the long term neutrality of money in this case? What would I need to change about my example, aside from adding cash, for him to agree with Scott? For example, would adding multiple commercial banks do it? Something else? What's the minimum change required?

Relating my hypothetical back to reality, is it really so outlandish to consider the case of a single commercial bank? Mark Sadowski identified a case in France in which the banks were nationalized, and he notes with little effect. In terms of a cashless society, Mark is convinced we'll still have cash a hundred years from now, but Scott agrees it is going away at some point. I believe Canada looked into the consequences of going cashless. But even if Mark is right and cash is here for the long haul, does it really make much of a difference? Isn't it just an arbitrary choice by the depositor about which form to keep his money in? (Mark & Mishkin agree it's the "depositor's choice.") Does it really affect the long term neutrality of money, the QTM and the HPE?

*Although only reserves are MOA, I think both Scott & Nick would agree that both reserves and commercial bank deposits are a medium of exchange (MOE) in this case.

For those wishing to see the above change in Ms sketched out in balance sheets, look here.

Additional notes and links can be found here (warning: redundant and unorganized!)

Although I compiled a more succinct history of this question here, (a copy here) and the question itself has been brought up again here and here. Also, I discuss the history of a preceding related question here (in which the CB buys up the commercial banks).